How to Turn a Improper Fraction to a Proper Fraction
Mixed Numbers and Improper Fractions
If you have a big pizza party and have one pineapple pizza and half an anchovy pizza left over afterward, you would say that you have "one and a half" pizzas. "One and a half" is the standard spoken-English way of expressing this number, and it is written as "11/2 ". This symbol, "11/2 ", is called a "mixed number", because it combines the "regular" number "1" with the fraction " 1/2 ".
While mixed numbers are the natural choice for spoken English (and are therefore well-suited to the answers of word problems), they aren't generally the easiest fractions to compute with. In algebra, you will almost always prefer that your fractions not be mixed numbers.
Content Continues Below
Instead, you will use so-called "improper" fractions (also sometimes called "vulgar" fractions), being fractions where the top number is bigger than the bottom number.
The standard way to convert a mixed number to an improper fraction is to multiply the bottom number by the "regular" number, add in the top number, and then put this on top of the original bottom number as a new fraction.
For instance, to convert 11/2 to an improper fraction, you do the following:
I multiplied the bottom 2 by the "regular" 1, and then added in the 1 from on top, getting 3. Then I put this 3 on top of the 2 from underneath.
-
Convert to an improper fraction.
To do the conversion, I'll multiply the denominator (the 16) by the whole number (the 2) to get 32. Then I'll add the numerator (the 3) to 32 to get the new numerator (35). The denominator will remain the same; namely, 16.
-
Convert to an improper fraction.
To do the conversion, I'll multiply the denominator (the 5) by the whole number (the 6) to get 30. Then I'll add the numerator (the 2) to 30 to get the new numerator (32). The denominator will remain the same; namely, 5.
To go from an improper fraction to a mixed number, you remember that "fractions are division", and you apply long division to find a whole-number quotient, plus a remainder. In other words, you divide the top number by the bottom number. Whatever you get on top of the division symbol is the quotient, and is your "regular number" part of the mixed number. Whatever your remainder is, put that number on top of the number you divided by; this is the fractional portion of your mixed number.
Note: When you're converting from improper fraction to mixed numbers, do not continue the long division into decimal places. Just find the quotient and the remainder. Then stop.
-
Convert to a mixed number.
First, I do the long division to find the whole-number part (being the quotient) and the remainder:
![]()
The quotient, across the top, is 11, so this will be the whole-number portion of the mixed number. Since the remainder is 1 and I'm dividing by 4, the fractional part will be 1/4 .
This procedure works perfectly well on rational expressions (polynomial fractions). You can see this in the example below (or else you can jump on ahead to multiplying regular fractions):
-
Convert to a mixed number.
First, do the long division to find the regular polynomial part and the remainder:
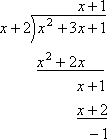
The polynomial on top is " x + 1" and the remainder is –1. Since you're dividing by " x + 2", the fractional part will be "(–1)/(x + 2)":
How to Turn a Improper Fraction to a Proper Fraction
Source: https://www.purplemath.com/modules/fraction2.htm